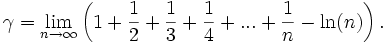Leonhard Euler
From Wikipedia, the free encyclopedia.

Leonhard Euler (IPA /ˈɔɪlər/) (April 15, 1707 – September 18, 1783) was a Swiss mathematician and physicist. He is considered to be one of the greatest mathematicians who ever lived. Leonhard Euler was the first to use the term "function" (defined by Leibniz in 1694) to describe an expression involving various arguments; i.e., y = F(x). He is credited with being one of the first to apply calculus to physics.
Born and educated in Basel, he was a mathematical child prodigy. He worked as a professor of mathematics in St. Petersburg, later in Berlin, and then returned to St. Petersburg. He is the most prolific mathematician of all time, his collected work filling 75 volumes. He dominated 18th century mathematics and deduced many consequences of the newly invented calculus. He was completely blind for the last seventeen years of his life, during which time he produced almost half of his total output.
The asteroid 2002 Euler is named in his honour.
Contents |
Biography

Leonhard Euler was born in Basel, Switzerland, the son of Paul Euler, a Lutheran minister. Although in his childhood he exhibited great mathematical talents, his father wanted him to study theology and become a minister. In 1720 Euler began his studies at the University of Basel. There Euler met Daniel and Nikolaus Bernoulli, who noticed Euler's skills in mathematics. Paul Euler had attended Jakob Bernoulli's mathematical lectures and respected his family. When Daniel and Nikolaus Bernoulli asked him to allow his son to study mathematics he finally agreed and Euler began to study mathematics.
In 1727 Euler was called to St. Petersburg by Catherine I of Russia and became professor of physics in 1730, with an additional mathematics appointment in 1733. Euler was the first to publish a systematic introduction to mechanics in 1736: Mechanica sive motus scientia analytice exposita ("Mechanics or motion explained with analytical science"—that is, calculus). In 1735 he lost much of his vision in the right eye due to excessive observation of the sun.
In 1733 he married Katharina Gsell, the daughter of the director of the academy of arts. They had thirteen children, of whom only three sons and two daughters survived. The descendants of these children, however, were in high positions in Russia in the 19th century.
In the year 1741 Euler became director of the mathematical class at the Prussian Academy of Sciences in Berlin. His time in Berlin was very productive; however, he did not have an easy position due to a lack of the king's favor. Therefore he returned to St. Petersburg in 1766, ruled by Catherine the Great at that time, and he remained there for the rest of his life.
Euler continued to be very productive, despite a complete loss of vision, due to his extraordinary powers of memory and mental calculation. It is reported that once he let his assistant calculate a series to 17 summands and noticed that his own result and the assistant's result differed in the 50th digit—a recalculation showed that Euler was right.
It has been calculated that it would take eight-hours work per day for 50 years to copy all his works by hand. It was not till the year 1910 that a collection of his complete works was published; it took about 70 volumes. It is reported by Legendre that often he would write down a complete mathematical proof between the first and the second call for supper.
Euler was a deeply religious Calvinist throughout his life. However, a widely told anecdote that says that Euler challenged Denis Diderot at the court of Catherine the Great with "Sir, (a+b)n/n = x; hence God exists, reply!" is false.
When Euler died, the mathematician and philosopher Marquis de Condorcet commented, "...et il cessa de calculer et de vivre" (and he ceased to calculate and to live).
Discoveries
Euler, with Daniel Bernoulli, established the law that the torque on a thin elastic beam is proportional to a measure of the elasticity of the material and the second moment of area of a cross section, about an axis through the center of mass and perpendicular to the plane of the moment, see Euler-Bernoulli beam equation.
He also deduced the Euler equations, a set of laws of motion in fluid dynamics, directly from Newton's laws of motion. These equations are formally identical to the Navier-Stokes equations with zero viscosity. They are interesting chiefly because of the existence of shock waves.
Euler made important contributions to the theory of differential equations. In particular, he is known for creating a series of approximations which are used in computational mechanics. The most famous of these approximations is known as Euler's method.
In number theory, Euler invented the totient function. The totient φ(n) of a positive integer n is defined to be the number of positive integers less than or equal to n and coprime to n. For example, φ(8) = 4 since the four numbers 1, 3, 5 and 7 are coprime to 8.
In mathematical analysis, it was Euler who synthesised Leibniz's differential calculus with Isaac Newton's method of fluxions.
Euler established his fame in 1735 by solving the long-standing Basel problem:
 ,
,
where ζ(s) is the Riemann zeta function.
He also showed the usefulness, consistency, and simplicity of defining the exponent of an imaginary number by means of the formula
This is Euler's formula, which establishes the central role of the exponential function. In essence, all functions studied in elementary analysis are either variations of the exponential function or they are polynomials. What Richard Feynman called "The most remarkable formula in mathematics" (more commonly called Euler's identity) is an easy consequence:
Also in 1735, Euler defined the Euler-Mascheroni constant useful for differential equations:
He is a co-discoverer of the Euler-Maclaurin formula which is an extremely useful tool for calculation of difficult integrals, sums, and series.
Euler wrote Tentamen novae theoriae musicae in 1739 which was an attempt to combine mathematics and music; a biography comments that the work was "for musicians too advanced in its mathematics and for mathematicians too musical".
In economics, Euler showed that if each factor of production is paid the value of its marginal product, then (under constant returns to scale) the total income and output will be completely exhausted.
In geometry and algebraic topology, there is a relationship (also called Euler's Formula) which relates the number of edges, vertices, and faces of a simply connected polyhedron. Given such a polyhedron, the sum of the vertices and the faces is always the number of edges plus two. i.e.: F - E + V = 2. The theorem also applies to any planar graph. For nonplanar graphs, there is a generalization: If the graph can be embedded in a manifold M, then F - E + V = χ(M), where χ is the Euler characteristic of the manifold, a constant which is invariant under continuous deformations. The Euler characteristic of a simply-connected manifold such as a sphere or a plane is 2. A generalization of Euler's formula for arbitrary planar graphs exists: F - E + V - C = 1, where C is the number of components in the graph.
In 1736 Euler solved, or rather proved insoluble, a problem known as the seven bridges of Königsberg, publishing a paper Solutio problematis ad geometriam situs pertinentis which was the earliest application of graph theory or topology.
The solution to the seven bridges problem reduced the land masses to points and the bridges to lines (or edges) connecting those points. Looking at how many lines came into a point gave that point a degree (a point with three lines touching it has a degree of three). An Euler circuit has all its points of even degree. This means it is possible to travel each line exactly once without retracing your steps and end at the same point in which you started. An Euler path has exactly two odd vertices. This means that it is possible to travel each line exactly once without retracing your steps, but you will not end where you began. The seven bridges problem is neither an Euler circuit nor Euler path. Hence, you cannot visit each of the bridges of Königsberg without retracing your steps.
Honours
Euler was ranked #77 on Michael H. Hart's list of the most influential figures in history.
Quotes
- "Read Euler, read Euler. He is the master of us all." —Pierre-Simon Laplace
Further reading
- Euler Leonhardt : "Lettres à une Princesse d'Allemagne " ; free book at : http://www.bookmine.org ;
- Euler, Leonhard (1748). Introductio in analysin infinitorum. English translation Introduction to Analysis of the Infinite by John Blanton (Book I, ISBN 0387968245, Springer-Verlag 1988; Book II, ISBN 0387971327, Springer-Verlag 1989).
- Dunham, William (1999). Euler: The Master of Us All, Washington: Mathematical Association of America. ISBN 0-88385-328-0.
- Heimpell, Hermann, Theodor Heuss, Benno Reifenberg (editors). 1956. Die großen Deutschen, volume 2, Berlin: Ullstein Verlag.
- Simmons, J. (1996). The giant book of scientists: The 100 greatest minds of all time, Sydney: The Book Company.
- Singh, Simon. (2000). Fermats letzter Satz, Munich: Deutscher Taschenbuch Verlag.
- Lexikon der Naturwissenschaftler, Spektrum Akademischer Verlag Heidelberg, 2000.
See also
- List of topics named after Leonhard Euler
- Leonhard Euler/EB1911 biography
- Mathematician
- Physicist
- Mathematical constants
- Complex number
- Euler's conjecture
- Euler's disk
- Euler's rotation theorem
- Euler's three-body problem
- Thirty-six officers problem
- Euler number
- Euler's totient function
- Child prodigy
- Eulerian path
- Euler diagram
- Euler-Lagrange equation
External links
- Biography at the MacTutor archive
- Euler Archive
- Fermat's Last Theorem Blog Covers topics in the history of Fermat's Last Theorem from Diophantus of Alexandria to Andrew Wiles.